Work Done by a Gravitational force
We next examine the work done on an object by a particular type of force - namely, the gravitational force acting on it. Figure 7-6 shows a particle-like tomato of mass 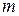 that is thrown upward with initial speed
that is thrown upward with initial speed 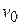 and thus with initial kinetic energy
and thus with initial kinetic energy 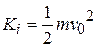 . As the tomato rises, it is slowed by a gravitational force
. As the tomato rises, it is slowed by a gravitational force 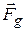 ; that is, the tomato's kinetic energy decreases because
; that is, the tomato's kinetic energy decreases because 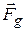 does work on the tomato as it rises.
does work on the tomato as it rises.
Because we can treat the tomato as a particle, we can use Eq. 7-7 ( 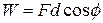 ) to express the work done during a displacement
) to express the work done during a displacement 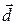 . For the force magnitude
. For the force magnitude 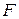 , we use
, we use  as the magnitude of
as the magnitude of 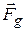 . Thus, the work
. Thus, the work 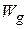 done by the gravitational force
done by the gravitational force 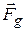 ,is
,is
 work done by gravitational force (7-12)
work done by gravitational force (7-12)
For a rising object, force 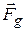 is directed opposite the displacement
is directed opposite the displacement 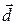 , as indicated in Fig. 7-6. Thus,
, as indicated in Fig. 7-6. Thus,  and
and
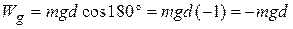 (7-13)
(7-13)
The minus sign tells us that during the object's rise, the gravitational force on the object transfers energy in the amount  from the kinetic energy of the object. This is consistent with the slowing of the object as it rises.
from the kinetic energy of the object. This is consistent with the slowing of the object as it rises.
After the object has reached its maximum height and is falling back down, the angle 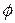 between force
between force 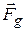 and displacement
and displacement 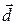 is zero. Thus,
is zero. Thus,
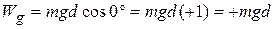
The plus sign tells us that the gravitational force now transfers energy in the amount  to the kinetic energy of the object. This is consistent with the speeding up of the object as it falls. (Actually, as we shall see in Chapter 8, energy transfers associated with lifting and lowering an object involve not just the object, but the full object-Earth system. Without Earth, of course, "lifting" would be meaningless.)
to the kinetic energy of the object. This is consistent with the speeding up of the object as it falls. (Actually, as we shall see in Chapter 8, energy transfers associated with lifting and lowering an object involve not just the object, but the full object-Earth system. Without Earth, of course, "lifting" would be meaningless.)
EXAMPLE 7—3 A child of weight  sits on a swing of length
sits on a swing of length 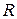 , as shown in Fig. 7-7. A variable horizontal force
, as shown in Fig. 7-7. A variable horizontal force 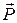 , which starts at zero and gradually increases, is used to pull the child very slowly (so that equilibrium exists at all times) until the ropes make an angle
, which starts at zero and gradually increases, is used to pull the child very slowly (so that equilibrium exists at all times) until the ropes make an angle 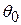 with the vertical. Calculate the work of the force
with the vertical. Calculate the work of the force 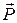 .
.
SOLUTION The body is in equilibrium, so the sum of the horizontal forces equals zero:
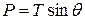 .
.
The sum of the vertical forces is also zero:
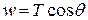 .
.
Dividing these two equations, we find
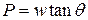 .
.
The point of application of 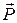 swings through the arcs. Since
swings through the arcs. Since 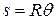 ,
,  and
and
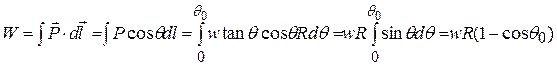
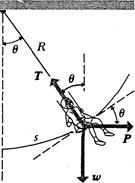
|
If  , there is no displacement; in that case
, there is no displacement; in that case  and
and  , as expected. If
, as expected. If  °, then
°, then 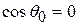 and
and 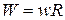 . In that case the work is the same as though the body had been lifted straight up a distance
. In that case the work is the same as though the body had been lifted straight up a distance 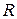 by a force equal to its weight
by a force equal to its weight  . In fact, as you should verify, the quantity
. In fact, as you should verify, the quantity  is the increase in the height of the body during the displacement; so for any value of
is the increase in the height of the body during the displacement; so for any value of 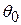 , the work done by force
, the work done by force 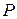 is the change in height multiplied by the weight. We will prove this result more generally in Section 7—5.
is the change in height multiplied by the weight. We will prove this result more generally in Section 7—5.
EXAMPLE 7—10 In Jules Verne's story "From the Earth to the Moon" (written in 1865) three men were shot to the moon in a shell fired from a giant cannon sunk in the earth in Florida. What muzzle velocity would be needed (a) to raise a total mass m to a height above the earth equal to the earth's radius; (b) to escape from the earth completely? To simplify the calculation, neglect the gravitational pull of the moon.
SOLUTION
a) In Eq. (7-23), let 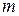 be the total projectile mass and let
be the total projectile mass and let 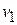 be the initial velocity.
be the initial velocity.
Then 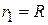 ,
,  ,
,  and
and
 or
or
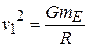

Then
b) When 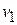 is the escape velocity,
is the escape velocity, 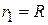 ,
,  ,
,  . Then
. Then
 or
or 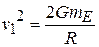 ,
,
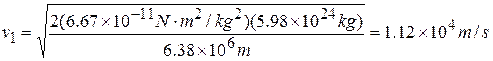
Note that the speed of an earth satellite in a circular orbit of radius just slightly greater than 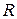 is, from Eq. (6—22), the same as the result of part (a), and that the escape velocity is larger than this by exactly a factor of
is, from Eq. (6—22), the same as the result of part (a), and that the escape velocity is larger than this by exactly a factor of 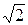 .
.
Дата добавления: 2015-06-17; просмотров: 820;
