Work Done in Lifting and Lowering an Object

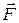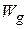Now suppose we lift a particle-like object by applying a vertical force to it. During the upward displacement, our applied force does positive work on the object while the gravitational force does negative work on it. Our force tends to transfer energy to the object while the gravitational force tends to transfer energy from it. By Eq. 7-10, the change 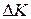in the kinetic energy of the object due to these two energy transfers is

(7-15)

in which 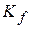is the kinetic energy at the end of the displacement and 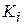is that at the start of the displacement. This equation also applies if we lower the object, but then the gravitational force tends to transfer energy to the object while our force tends to transfer energy from it.

In one common situation the object is stationary before and after the lift - for example, when you lift a book from the floor to a shelf. Then 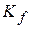and 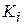are both zero, and Eq. 7-15 reduces to

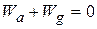
Or

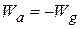(7-16)

Note that we get the same result if 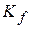and 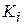are not zero but are still equal. Either way, the result means that the work done by the applied force is the negative of the work done by the gravitational force; that is, the applied force transfers the same amount of energy to the object as the gravitational force transfers from the object. Using Eq. 7-12, we can rewrite Eq. 7-16 as

(work in lifting and lowering; 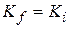(7-17)

with 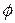being the angle between 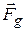and 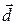. If the displacement is vertically upward (Fig. 7-7 a), then and the work done by our force equals . If the displacement is vertically downward (Fig. 1-lb), then 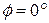and the work done by the applied force equals .

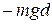Equations 7-16 and 7-17 apply to any situation in which an object is lifted or lowered, with the object stationary before and after the lift. They are independent of the magnitude of the force used. For example, when Chemerkin made his record-breaking lift, his force on the object he lifted varied considerably during the lift. Still, because the object was stationary before and after the lift, the work he did is given by Eqs. 7-16 and 7-17, where, in Eq. 7-17, mg is the weight of the object he lifted and d is the distance he lifted it.