Proof of Eqs. 11-44 through 11-47
Let us again consider the situation of Fig. 11-16, in which force 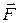 rotates a rigid body consisting of a single particle of mass
rotates a rigid body consisting of a single particle of mass 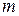 fastened to the end of a massless rod. During the rotation, force
fastened to the end of a massless rod. During the rotation, force 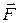 does work on the body. Let us assume that the only energy of the body that is changed by
does work on the body. Let us assume that the only energy of the body that is changed by 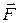 is the kinetic energy. Then we can apply the work-kinetic energy theorem of Eq. 11-41
is the kinetic energy. Then we can apply the work-kinetic energy theorem of Eq. 11-41
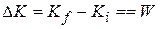 . 11-48
. 11-48
Using 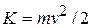 and Eq 11-18 (
and Eq 11-18 ( 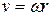 ) we can rewrite Eq. 11-48 as
) we can rewrite Eq. 11-48 as
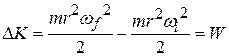
(11-
From Eq. 11-26, the rotational inertia for this one-particle body is 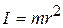 . Substituting this into Eq. 11-49 yields
. Substituting this into Eq. 11-49 yields
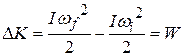 ,
,
which is Eq. 11-44. We derived it for a rigid body with one particle, but it holds for any rigid body rotated about a fixed axis.
We next relate the work 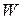 done on the body in Fig. 11-16 to the torque
done on the body in Fig. 11-16 to the torque 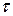 оп the body due to force
оп the body due to force 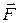 . When the particle moves a distance
. When the particle moves a distance 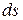 along its circular path, only the tangential component Ft of the force accelerates the particle along the path. Therefore, only
along its circular path, only the tangential component Ft of the force accelerates the particle along the path. Therefore, only  does work on the particle. We write that work
does work on the particle. We write that work 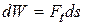 . However, we can replace
. However, we can replace 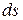 with
with 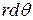 , where
, where 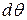 is the angle through which the particle moves. Thus we have
is the angle through which the particle moves. Thus we have
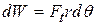 . (11-50)
. (11-50)
From Eq. 11-32, we see that the product 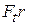 is equal to the torque
is equal to the torque 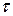 , so we can rewrite Eq. 11-50 as
, so we can rewrite Eq. 11-50 as
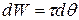 (11-51)
(11-51)
The work done during a finite angular displacement from 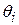 to
to 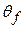
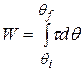
which is Eq. 11-45. It holds for any rigid body rotating about a fixed axis. Equation 11-46 comes directly from Eq. 11-45.
We can find the power P for rotational motion from Eq. 11-51:
which is Eq. 11-47
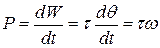
Дата добавления: 2015-06-17; просмотров: 594;
