Kinematics
MECHANICS
Our study of physics begins in the area of mechanics, which deals with the relations among force, matter, and motion. Mechanics consists of: kinematics, dynamics and static.
Kinematics is the study of motion of objects without taking into account the causes of their motion.
Dynamics is the study of motion of objects by taking into account the cause of their change of state (rest or of uniform motion).
Static is study of the object at rest under the effect of forces in equilibrium.
Motion is a continuous change of the position of a body with respect to its surroundings
In physics we are concerned with three types of motion: translational, rotational, and vibrational. A car moving down a highway is an example of translational motion, the Earth's spin on its axis is an example of rotational motion, and the back-and-forth movement of a pendulum is an example of vibrational motion.
In our study of translational motion, we describe the moving object as a particle regardless of its size. In general a particle is a point-like mass having infinitesimal size. For example, if we wish to describe the motion of the Earth around the Sun, we can treat the Earth as a particle and obtain reasonably accurate data about its orbit. Wesay that the particle is a model for a moving body; it provides a simplified, idealized description of the position and motion of the body.
1-1 Position and Displacement
To locate an object means to find its position relative to some reference point, often the origin(or zero point) of an axis such as the  axis in Fig. 2-1. The positive directionof the axis is in the direction of increasing numbers (coordinates), which is toward the right in Fig. 1. The opposite direction is the negative direction.
axis in Fig. 2-1. The positive directionof the axis is in the direction of increasing numbers (coordinates), which is toward the right in Fig. 1. The opposite direction is the negative direction.
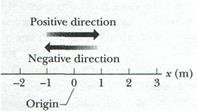 Fig. 1-1
Fig. 1-1
|
For example, a particle might be located at 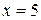 m, which means that it is 5 m in the positive direction from the origin. If it were at
m, which means that it is 5 m in the positive direction from the origin. If it were at  m, it would be just as far from the origin but in the opposite direction.
m, it would be just as far from the origin but in the opposite direction.
A change from one position 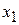 to another position
to another position 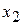 is called a displacement
is called a displacement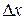 , where
, where
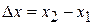 (1)
(1)
(The symbol 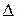 , the Greek uppercase delta, represents a change in a quantity, and it means the final value of that quantity minus the initial value.) When numbers are inserted for the position values
, the Greek uppercase delta, represents a change in a quantity, and it means the final value of that quantity minus the initial value.) When numbers are inserted for the position values 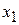 and
and 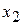 , a displacement in the positive direction (toward the right in Fig. 1) always comes out positive, and one in the opposite direction (left in the figure), negative. For example, if the particle moves from
, a displacement in the positive direction (toward the right in Fig. 1) always comes out positive, and one in the opposite direction (left in the figure), negative. For example, if the particle moves from 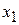 = 5m to
= 5m to 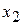 = 12 m, then
= 12 m, then 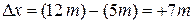 . The positive result indicates that the motion is in the positive direction. If the particle then returns to
. The positive result indicates that the motion is in the positive direction. If the particle then returns to 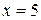 m, the displacement for the full trip is zero. The actual number of meters covered for the full trip is irrelevant; displacement involves only the original and final positions.
m, the displacement for the full trip is zero. The actual number of meters covered for the full trip is irrelevant; displacement involves only the original and final positions.
A plus sign for a displacement need not be shown, but a minus sign must always be shown. If we ignore the sign (and thus the direction) of a displacement, we are left with the magnitude(or absolute value) of the displacement. In the previous example, the magnitude of 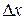 is 7 m.
is 7 m.
Displacement is an example of a vector quantity,which is a quantity that has both a direction and a magnitude. Here all we need is the idea that displacement has two features: (1) Its magnitude is the distance (such as the number of meters) between the original and final positions. (2) Its direction, from an original position to a final position, can be represented by a plus sign or a minus sign if the motion is along a single axis.
•CHECKPOINT 1:Here are three pairs of initial and final positions, respectively, along an  axis. Which pairs give a negative displacement: (a)
axis. Which pairs give a negative displacement: (a) 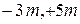 ; (b)
; (b) 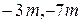 ; (c)
; (c) 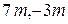 ?
?
Exercises
1. A watermelon seed has the following coordinates: 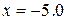 m,
m, 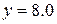 m, and
m, and 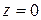 m. Find its position vector (a) in unit-vector notation and as (b) a magnitude and (c) an angle relative to the positive direction of the
m. Find its position vector (a) in unit-vector notation and as (b) a magnitude and (c) an angle relative to the positive direction of the  axis, (d) Sketch the vector on a right-handed coordinate system. If the seed is moved to the xyz coordinates (3.00 m, 0 m, 0 m), what is its displacement (e) in unit-vector notation and as (f) a magnitude and (g) an angle relative to the positive direction of the x axis?
axis, (d) Sketch the vector on a right-handed coordinate system. If the seed is moved to the xyz coordinates (3.00 m, 0 m, 0 m), what is its displacement (e) in unit-vector notation and as (f) a magnitude and (g) an angle relative to the positive direction of the x axis?
2. The position vector for an electron is  . (a) Find the magnitude of
. (a) Find the magnitude of  . (b) Sketch the vector on a right-handed coordinate system.
. (b) Sketch the vector on a right-handed coordinate system.
3, The position vector for a proton is initially 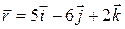 and then later is
and then later is 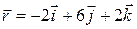 , all in meters. (a) What is the proton's displacement vector, and (b) to what plane is that vector parallel?
, all in meters. (a) What is the proton's displacement vector, and (b) to what plane is that vector parallel?
1-2 Average Velocity and Average Speed
A compact way to describe position is with a graph of position  plotted as a function of time
plotted as a function of time 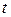 - a graph of
- a graph of 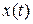 . (The notation
. (The notation  represents a function
represents a function 
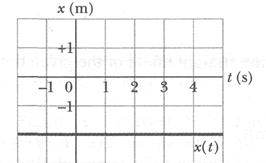 Fig. 1-2
Fig. 1-2
|
of  , not the product
, not the product  times
times  .)As a simple example, Fig. 1-2 shows the position function
.)As a simple example, Fig. 1-2 shows the position function 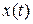 for a stationary armadillo (which we treat as a particle) at
for a stationary armadillo (which we treat as a particle) at 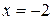 m.
m.
Fig.2-2. The graph of 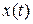 for an armadillo that is stationary at
for an armadillo that is stationary at 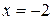 m. The value of
m. The value of  is
is 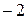 m for all times
m for all times 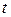
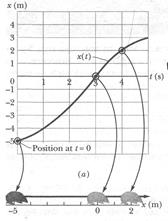 Figure 1-3a, b
Figure 1-3a, b
|
Figure 1-3a, also for an armadillo, is more interesting, because it involves motion. The armadillo is apparently first noticed at 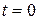 when it is at the position
when it is at the position  m. It moves toward
m. It moves toward 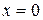 , passes through that point at
, passes through that point at 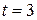 s, and then moves on to increasingly larger positive values of
s, and then moves on to increasingly larger positive values of 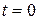 .
.
Figure 1-3bdepicts the actual straight-line motion of the armadillo and is something like what you would see. The graph in Fig. 1-3ais more abstract and quite unlike what you would see, but it is richer in information. It also reveals how fast the armadillo moves.
Actually, several quantities are associated with the phrase "how fast." One of them is the average velocity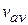 , which is the ratio of the displacement
, which is the ratio of the displacement 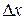 : that occurs during a particular time interval
: that occurs during a particular time interval 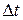 to that interval^
to that interval^
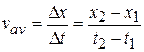 (1-2)
(1-2)
The notation means that the position is 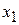 at time
at time 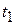 and then
and then 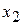 at time
at time 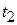 . A common unit for
. A common unit for 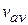 is the meter per second (m/s). You may see other units in the problems, but they are always in the form of length/time.
is the meter per second (m/s). You may see other units in the problems, but they are always in the form of length/time.
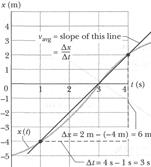 Figure 1-4
Figure 1-4
|
On a graph of  versus
versus 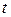 ,
, 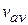 is the slopeof the straight line that connects two particular points on the
is the slopeof the straight line that connects two particular points on the 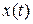 curve: one is the point that corresponds to
curve: one is the point that corresponds to 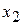 and
and 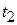 , and the other is the point that corresponds to
, and the other is the point that corresponds to 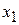 and
and 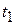 . Like displacement,
. Like displacement, 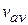 has both magnitude and direction (it is another vector quantity). Its magnitude is the magnitude of the line's slope. A positive
has both magnitude and direction (it is another vector quantity). Its magnitude is the magnitude of the line's slope. A positive 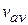 (and slope) tells us that the line slants upward to the right; a negative
(and slope) tells us that the line slants upward to the right; a negative 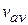 (and slope), that the line slants downward to the right. The average velocity
(and slope), that the line slants downward to the right. The average velocity 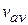 always has the same sign as the displacement
always has the same sign as the displacement 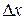 because
because 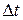 in Eq. 1-2 is always positive. Figure 1-4 shows how to find
in Eq. 1-2 is always positive. Figure 1-4 shows how to find 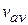 for the armadillo of Fig. 1-3, for the time interval
for the armadillo of Fig. 1-3, for the time interval 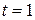 s to
s to 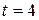 s. We draw the straight line that connects the point on the position curve at the beginning of the interval and the point on the curve at the end of the interval. Then we find the slope
s. We draw the straight line that connects the point on the position curve at the beginning of the interval and the point on the curve at the end of the interval. Then we find the slope 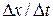 of the straight line. For the given time interval, the average velocity is
of the straight line. For the given time interval, the average velocity is
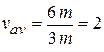 m/s.
m/s.
Average speed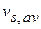 is a different way of describing "how fast" a particle moves. Whereas the average velocity involves the particle's displacement
is a different way of describing "how fast" a particle moves. Whereas the average velocity involves the particle's displacement 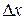 , the average speed involves the total distance covered (for example, the number of meters moved), independent of direction; that is,
, the average speed involves the total distance covered (for example, the number of meters moved), independent of direction; that is,
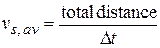
Because average speed does not include direction, it lacks any algebraic sign. Sometimes 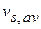 is the same (except for the absence of a sign) as
is the same (except for the absence of a sign) as 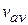 . However, as is demonstrated in Sample Problem 1-1, when an object doubles back on its path, the two can be quite different.
. However, as is demonstrated in Sample Problem 1-1, when an object doubles back on its path, the two can be quite different.
Example 1-1
You drive a beat-up pickup truck along a straight road for 8.4 km at 70 km/h, at which point the truck runs out of gasoline and stops. Over the next 30 min, you walk another 2.0 km farther along the road to a gasoline station.
(a) What is your overall displacement from the beginning of your
drive to your arrival at the station?
Solution. Assume, for convenience, that you move in the positive direction of an  axis, from a first position of
axis, from a first position of 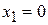 to a second position of
to a second position of 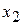 at the station. That second position must be at
at the station. That second position must be at  km. Then your displacement
km. Then your displacement 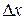 along the
along the  axis is the second position minus the first position. From Eq. 1-1, we have
axis is the second position minus the first position. From Eq. 1-1, we have
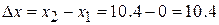 km. (Answer)
km. (Answer)
Thus, your overall displacement is 10.4 km in the positive direction of the  axis.
axis.
(b) What is the time interval 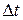 from the beginning of your drive
from the beginning of your drive
to your arrival at the station?
Solution. We already know the time interval 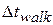 (= 0.50 h) for the walk, but we lack the time interval
(= 0.50 h) for the walk, but we lack the time interval 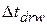 for the drive. However, we know that for the drive the displacement
for the drive. However, we know that for the drive the displacement  is 8.4 km and the average velocity
is 8.4 km and the average velocity 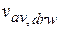 is 70 km/h. A Key Idea to use here comes from Eq. 1-2: This average velocity is the ratio of the displacement for the drive to the time interval for the drive:
is 70 km/h. A Key Idea to use here comes from Eq. 1-2: This average velocity is the ratio of the displacement for the drive to the time interval for the drive:
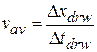
Rearranging and substituting data then give us
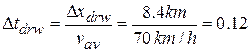 h.
h.
So, 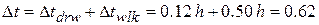 h. (Answer)
h. (Answer)
(c) What is your average velocity 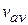 from the beginning of your drive to your arrival at the station? Find it both numerically and graphically.
from the beginning of your drive to your arrival at the station? Find it both numerically and graphically.
Solution. The Key Idea here again comes from Eq. 1-2: 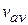 for the entire trip is the ratio of the displacement of 10.4 km for the entire trip to the time interval of 0.62 h for the entire trip. With Eq. 1-2, we find it is
for the entire trip is the ratio of the displacement of 10.4 km for the entire trip to the time interval of 0.62 h for the entire trip. With Eq. 1-2, we find it is
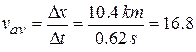 km/h (Answer)
km/h (Answer)
To find 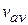 graphically, first we graph
graphically, first we graph 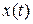 as shown in Fig. 1-5, where the beginning and arrival points on the graph are the origin and the point labeled as "Station." The Key Idea here is that your average velocity is the slope of the straight line connecting those points; that is, it is the ratio of the rise (
as shown in Fig. 1-5, where the beginning and arrival points on the graph are the origin and the point labeled as "Station." The Key Idea here is that your average velocity is the slope of the straight line connecting those points; that is, it is the ratio of the rise ( 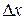 = 10.4 km) to the run (
= 10.4 km) to the run ( 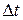 = 0.62 h), which gives us
= 0.62 h), which gives us 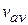 = 16.8 km/h.
= 16.8 km/h.
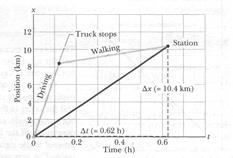 Fig. 1-5
Fig. 1-5
|
(d) Suppose that to pump the gasoline, pay for it, and walk back to the truck takes you another 45 min. What is your average speed from the beginning of your drive to your return to the truck with the gasoline?
Solution.: The Key Idea here is that your average speed is the ratio of the total distance you move to the total time interval you take to make that move. The total distance is 8.4 km + 2.0 km + 2.0 km = 12.4 km. The total time interval is 0.12 h + 0.50 h + 0.75 h = 1.37 h. Thus, Eq. 2-3 gives us
 km/h (Answer)
km/h (Answer)
1-4 Instantaneous Velocity and Speed
You have now seen two ways to describe how fast something moves: average velocity and average speed, both of which are measured over a time interval 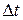 . However, the phrase "how fast" more commonly refers to how fast a particle is moving at a given instant - and that is its instantaneous velocity(or simply velocity)
. However, the phrase "how fast" more commonly refers to how fast a particle is moving at a given instant - and that is its instantaneous velocity(or simply velocity) .
.
The velocity at any instant is obtained from the average velocity by shrinking the time interval 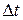 closer and closer to 0. As
closer and closer to 0. As 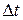 dwindles, the average velocity approaches a limiting value, which is the velocity at that instant:
dwindles, the average velocity approaches a limiting value, which is the velocity at that instant:
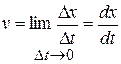 (1-4)
(1-4)
This equation displays two features of the instantaneous velocity  . First,
. First,  is the rate at which the particle's position
is the rate at which the particle's position  is changing with time at a given instant; that is,
is changing with time at a given instant; that is,  is the derivative of
is the derivative of  with respect to
with respect to 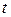 . Second,
. Second,  at any instant is the slope of the particle's position-time curve at the point representing that instant. Velocity is another vector quantity and thus has an associated direction.
at any instant is the slope of the particle's position-time curve at the point representing that instant. Velocity is another vector quantity and thus has an associated direction.
Speedis the magnitude of velocity; that is, speed is velocity that has been stripped of any indication of direction, either in words or via an algebraic sign. A velocity of +5 m/s and one of -5 m/s both have an associated speed of 5 m/s. The speedometer in a car measures the speed, not the velocity, because it cannot determine the direction.
Example 2-3
The position of a particle moving on an  axis is given by
axis is given by
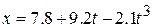
with  in meters and
in meters and 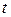 in seconds. What is its velocity at
in seconds. What is its velocity at 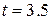 s? Is the velocity constant, or is it continuously changing?
s? Is the velocity constant, or is it continuously changing?
Solution. For simplicity, the units have been omitted from Eq. 1-5, but you can insert them if you like by changing the coefficients to 7.8 m, 9.2 m/s, and -2.1 m/s3. The Key Idea here is that velocity is the first derivative (with respect to time) of the position function 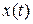 . Thus, we write
. Thus, we write
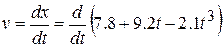
which becomes
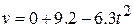 1-6 (Answer)
1-6 (Answer)
At 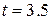 s, the particle is moving in the negative direction of
s, the particle is moving in the negative direction of  (note the minus sign) with a speed of 68 m/s. Since the quantity
(note the minus sign) with a speed of 68 m/s. Since the quantity 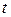 appears in Eq. 1-6, the velocity
appears in Eq. 1-6, the velocity  depends on
depends on 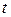 and so is continuously changing.
and so is continuously changing.
Exercises
1.5 A hobbyist is testing a new model rocket engine by using it to propel a cart along a model railroad track. He determines that its motion along the  -axis is described by the equation
-axis is described by the equation 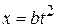 , where
, where 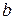 = 10 cm/s2. Compute the instantaneous velocity of the cart at time
= 10 cm/s2. Compute the instantaneous velocity of the cart at time 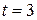 s.
s.
1.6 A car is initially stopped at a traffic light. It then travels along a straight road such that its distance from the light is given by 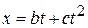 , where b= 4.0 m/s and с = 0.5 m/s2.
, where b= 4.0 m/s and с = 0.5 m/s2.
a) Calculate the average velocity of the car for the time interval 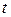 = 0to
= 0to 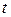 =10s.
=10s.
b)Calculate the instantaneous velocity of the car at i) t =0; ii) t = 5 s; iii) t =10 s
1-5 Acceleration
When a particle's velocity changes, the particle is said to undergo acceleration (or to accelerate). For motion along an axis, the average acceleration 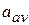 over a time interval
over a time interval 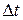 is
is
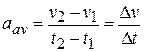 (1-7)
(1-7)
where the particle has velocity 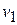 at time
at time 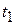 and then velocity
and then velocity 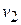 at time
at time 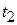 . The instantaneous acceleration(or simply acceleration)is the derivative of the velocity with respect to time:
. The instantaneous acceleration(or simply acceleration)is the derivative of the velocity with respect to time:
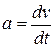 (1-8)
(1-8)
In words, the acceleration of a particle at any instant is the rate at which its velocity is changing at that instant. Graphically, the acceleration at any point is the slope of the curve of 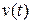 at that point.
at that point.
We can combine Eq. 1-8 with Eq. 1-4 to write
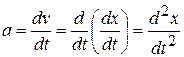 (1-9)
(1-9)
In words, the acceleration of a particle at any instant is the second derivative of its position 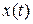 with respect to time.
with respect to time.
A common unit of acceleration is the meter per second per second: m/(s • s) or m/s2. You will see other units in the problems, but they will each be in the form of length/(time • time) or length/time2. Acceleration has both magnitude and direction (it is yet another vector quantity). Its algebraic sign represents its direction on an axis just as for displacement and velocity; that is, acceleration with a positive value is in the positive direction of an axis, and acceleration with a When  is constant, the derivative is zero and so also is the acceleration.
is constant, the derivative is zero and so also is the acceleration.
Your body reacts to accelerations (it is an accelerometer) but not to velocities (it is not a speedometer). When you are in a car traveling at 90 km/h or an airplane traveling at 900 km/h, you have no bodily awareness of the motion. However, if the car or plane quickly changes velocity, you may become keenly aware of the change, perhaps even frightened by it.
Large accelerations are sometimes expressed in terms of 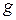 units, with
units, with
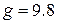 m/s2
m/s2
 Example 1-4
Example 1-4
A particle's position on the  axis of Fig. 1-1 is given by
axis of Fig. 1-1 is given by
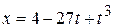 , with
, with  in meters and
in meters and 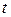 in seconds.
in seconds.
a) Find the particle's velocity function  and acceleration function
and acceleration function  .
.
Solution. One Key Idea is that to get the velocity function  , we differentiate the position function
, we differentiate the position function  with respect to time. Here we find
with respect to time. Here we find
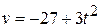
with  in meters per second.
in meters per second.
Another Key Idea is that to get the acceleration function  , we differentiate the velocity function
, we differentiate the velocity function  with respect to time. This gives us
with respect to time. This gives us
 , (Answer)
, (Answer)
with 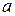 in meters per second squared.
in meters per second squared.
(b) Is there ever a time when 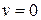 ?
?
Solution. Setting 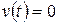 yields
yields
0 = -27 + 3t2, which has the solution
t = ±3 s. (Answer)
Thus, the velocity is zero both 3 s before and 3 s after the clock reads 0.
(c) Describe the particle's motion for 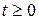 .
.
Solution. The Key Idea is to examine the expressions for  ,
,  , and
, and  .
.
At 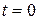 , the particle is at
, the particle is at 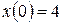 m and is moving with a velocity of
m and is moving with a velocity of 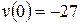 m/s - that is, in the negative direction of the
m/s - that is, in the negative direction of the  axis. Its acceleration is
axis. Its acceleration is 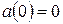 , because just then the particle's velocity is not changing.
, because just then the particle's velocity is not changing.
For 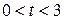 s, the particle still has a negative velocity, so it continues to move in the negative direction. However, its acceleration is no longer 0 but is increasing and positive. Because the signs of the velocity and the acceleration are opposite, the particle must be slowing.
s, the particle still has a negative velocity, so it continues to move in the negative direction. However, its acceleration is no longer 0 but is increasing and positive. Because the signs of the velocity and the acceleration are opposite, the particle must be slowing.
Indeed, we already know that it stops momentarily at 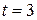 s. Just then the particle is as far to the left of the origin in Fig. 2-1 as it will ever get. Substituting
s. Just then the particle is as far to the left of the origin in Fig. 2-1 as it will ever get. Substituting 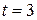 s into the expression for
s into the expression for  , we find that the particle's position just then is
, we find that the particle's position just then is 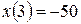 m. Its acceleration is still positive.
m. Its acceleration is still positive.
For 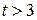 s, the particle moves to the right on the axis. Its acceleration remains positive and grows progressively larger in magnitude. The velocity is now positive, and it too grows progressively larger in magnitude.
s, the particle moves to the right on the axis. Its acceleration remains positive and grows progressively larger in magnitude. The velocity is now positive, and it too grows progressively larger in magnitude.
Exercises
1.33 A motorcycle's position is described by 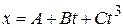 x = A + Bt + Cts, where A, B, and С are numerical constants.
x = A + Bt + Cts, where A, B, and С are numerical constants.
a)Calculate the velocity of the cycle, as a function of time.
b) From your answer to (a), calculate the acceleration of the motorcycle as a function of time.
1.. Are rest and motion absolute or relative terms
1.02. Under what condition will the distance and displacement of a moving object will have the same magnitude?
1..03. What will be nature of  graph for a uniform motion?
graph for a uniform motion?
1.04. Can  graph be a straight line parallel to time-axis?
graph be a straight line parallel to time-axis?
1.05. Can  graph be a straight line parallel to position-axis?
graph be a straight line parallel to position-axis?
1.06. What does slope of  graph represent?
graph represent?
1.07. Can  graph have a negative slope?
graph have a negative slope?
1..08. Can a particle in one dimensional motion with zero speed may have non-zero velocity
1..09. Can a body have a constant velocity but a varying speed?
1..10. Can a body have a constant speed but a varying velocity? Or Is it possible for a body to be accelerated, if its speed is constant ? If it is so, give an example.
Ans. Yes. When a body moves along a circular path with/ uniform angular speed, it possesses constant speed but a varying velocity.
1..11. What does the speedometer of a car measure?
1..12. When will the relative velocity of two moving objects be zero?
1..06 Is magnitude of the displacement of an object and the total distance covered by it in certain time interval same? Explain.
1. A cyclist moves along a circular path of radius 70 m. If he completes one round in 11 s, calculate (i) total length of path, (ii) magnitude of the displacement, (iii) average speed and (iv) magnitude of average velocity.
[Ans. (i) 440 m ; (it) zero ; (iii) 40 m s_1; (iv) zero]
Дата добавления: 2015-06-17; просмотров: 758;
