Models for the description of continuous systems
The linear differential equations (DE) it is the most general form of the description of dynamics, however their practical use and engineering practice often can be difficult.
The most general view of record DE:
| a | d n x | + ...+ a | dx | + a x = b | d mu | + ...+ b | du | + b u (2.3). | |||
| n+1 dt n | 2 dt | ||||||||||
| m+1 dt m | 2 dt | ||||||||||
Transfer Functions (TF). In engineering practice MMD in ви are often used by PF. For objects with self-alignment of PF in a general view:
W ( p) = C Ч b1 + b2 Ч p + + bm+1 Ч pm Ч exp(- p Чt ) . (n≥m) (2.4)
 a1+ a2Ч p + + an+1 Ч pn
a1+ a2Ч p + + an+1 Ч pn
When using "a method of the areas" (b1=a1=1) []:
| W ( p)= C Ч | b1+ b2Ч p | Ч exp(- p Чt ) or (2.5) | ||||||||||||||
| a | + a | Ч p + a | Ч p 2 | |||||||||||||
| W ( p)= C Ч | b1 | Ч exp(- p Чt ) (2.6) | ||||||||||||||
| a | + a | Ч p + a | Ч p 2 | + a | Ч p3 | |||||||||||
| Other types, for example: | ||||||||||||||||
| W ( p)= | C Чexp(- p Чt ) | etc. (2.7) | ||||||||||||||
| n | ||||||||||||||||
| Х(1 + Ti Ч p) | ||||||||||||||||
i =1
Very often apply the simplified expression of PF in the form of an aperiodic link with delay:
| W ( p)= | C | Ч exp(- p Чt ) | (2.8) |
| 1 + T Ч p |

For objects without self-alignment transfer function in the general case is used used in a look:
| йD | b + b Ч p + + b | Ч pm щ | ||||||
| W ( p)= к | - C Ч | m+1 | ъ | Чexp(- p Чt) (2.9) | ||||
| к p | a + a | Ч p + + a | n+1 | Ч pn ъ | ||||
| л | ы |
(we pay attention that there is an integrated component - size 1/p) or the simplified expressions for objects without self-alignment:
| W ( p)= | C | Ч exp(- p Чt ) or: | (2.10) | |||
| p Ч TБ Ч(1+ T Ч p) | ||||||
| W ( p)= | C | Ч exp(- p Чt ) | (2.11) | |||
| T Ч | p |
The concrete type of W(p) gets out of a condition of ensuring adequacy and convenience of calculations.
Adequacy of mathematical modelcan be estimated, for example, on a formula (2.12). Ifthe value d found on this formula doesn't exceed 3-7%, then the model is considered adequate:
| N | - | Щ | |||||
| 100% | X | i | X i | ||||
| d = | Ч е | ||||||
| N | X | i | |||||
| i =1 | |||||||
Щ
For the equation of a look (2.8) an exit X i example in the figure 8.7):
| Щ | й | t -t | щ | |||
| X (t)= C | Ч DU Чк1 | - exp(- | )ъ | (for | ||
| Щ | л | T | ы | |||
| <t ) (2.13A) | ||||||
| X (t)=0(for | t |
(2.12)
is determined analytically by a formula (see an
t іt ) (2.13)
and for W(p) of a look (2.7) at n=2: W ( p)
| Щ | ж | T | t -t | |||
| X (t)= C Ч DU Ч з1 | - | Ч exp(- | ||||
| з | T1- T2 | T1 | ||||
| и |
| = | C Чexp(- p Чt ) | |||||
| (1 + T1 Ч p) Ч (1 + T2 Ч p) | ||||||
| T | t -t | ц | ||||
| ) + | Ч exp(- | ) ч (2.14) | ||||
| T1- T2 | T2 | ч | ||||
| ш |
In more general case as criterion of compliance at the solution of this task take criterion of a look:
| n ж | Щ ц2 | |
| з | ч | (2.15) |
| min(Fai), F (ai ) = ез X i - X i ч | ||
| j =1и | ш |
where X i – experimental value;
Щ
X i –calculated value.
For finding of coefficients of ai work out the equations:
| ¶F | = 0 | (2.16) |
| ¶ai |
Thus, turns out the system of the equations, solving which it is possible to define ai. Adequacy of the received mathematical models of a statics can be checked was checked by
Fischer [1] criterion (see also next lecture). In addition so-called criteria of suitability of approach [5] the R-square (determination coefficient) used for Estimate of accuracy of nonlinear models can be for this purpose used more convenient. Criterion a R-square he can accept values only from zero to unit and the closer to unit, the better the parametrical model brings closer basic data.
For his definition the criterion of SSE (Sum of squares due to error) - the sum of squares of errors on a formula is calculated in the beginning:
n
SSE =еwk Ч( yk - y€k )2
k =1
where wk - weight (at us they aren't set, and are considered equal to unit); yk - experimental (initial) values of data for each experience;
y€k - the calculated (predicted) values of data for each experience, are received on a
formula (1);
n - amount of experimental values (for example, n=20).
The criterion the R-square (designated below as R) is defined as the relation of the sum of squares concerning regression of SSR to the full sum of squares (SST), i.e.:
| n | n | SSR | SSE | |||||
| SSR =еwk Ч( y€k - | SST =еwk Ч( yk - | R = | =1 - | , | ||||
| SST | SST | |||||||
| k =1 | k =1 | |||||||
| where | ||||||||
| The proximity of the received values of criterion tells a R-square to unit about high precision | ||||||||
| of the description of an experiment, for example expression of a look (2.2). Usually accepted for | ||||||||
| practice consider values of criterion a R-square higher than 0,9. | ||||||||
| This indicator is a statistical measure of a consent. The coefficient of determination changes | ||||||||
| in the range from 0 to 1. If he is equal 0, it means that communication between variables of | ||||||||
| regression model is absent. On the contrary, if the coefficient of determination is equal 1, it | ||||||||
| corresponds to ideal model when all points of observations lie precisely on the line of regression, | ||||||||
| i.e. the sum of squares of their deviations is equal to 0. | ||||||||
| Sometimes indicators of narrowness of communication can give quality standard (Cheddok's | ||||||||
| scale): | ||||||||
| Quantitative measure of narrowness of communication Qualitative characteristic of force of | ||||||||
| communication | ||||||||
| 0,1 | - 0,3 | Weak | ||||||
| 0,3 | - 0,5 | Moderate | ||||||
| 0,5 | - 0,7 | Noticeable | ||||||
| 0,7 | - 0,9 | High | ||||||
| 0,9 | - 0,99 | Very high |
Functional communication arises at value equal 1, and lack of communication — 0. At values of indicators of narrowness of communication less than 0,7 size of coefficient of determination will always be lower than 50%.
Frequency Characteristics (FC). When giving on an entrance of linear system of a signal:
| U (t)= Au sin(wt)= Au Чexp( jwt) | (2.17) |
at the exit there will be a signal:
| X (t)= Ax sin(wt + j)= Ax Чexp( jwt + j) | (2.18) | ||||||||||
| and APFC has an appearance: | |||||||||||
| b + b Ч jw + + b | Ч jwm | ||||||||||
| W ( jw)= C Ч | m+1 | Чexp(- jw Чt ) | = | ||||||||
| Ч jwn | |||||||||||
| a + a | Ч jw + + a | n+1 | |||||||||
| = A(w)Чexp( jf(w))=ReW (w)+ImW (w) | |||||||||||
| (2.19), | |||||||||||
| Where: | |||||||||||
| A(w)= | [ReW (w)]2 + [ImW (w)]2 | (2.20) | |||||||||
| [ImW (w)] | |||||||||||
| j(w) = arctan [ReW (w)] | (2.21) |
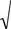
Also weight functions are used:
| t | t | |
| x(t)=т g(t -t ) y(t )dt =т g(t ) y(t -t )dt | ||
| (2.22) |
And systems of matrix linear equations of space of a status:
Model in space of parameters of a status
x = Ax + bU
| y = CT x | (2.23) |
where
U - input vector; x - a vector of state variables; y - system output vector;
A - a matrix of dynamics of system; B - a control matrix; CT - a matrix of measurement (sensors)
or
| x = AЧ x(t)+ B Чu(t); | |
| y(t)= C Ч x(t)+ D Чu(t) | (2.24) |
Дата добавления: 2017-05-18; просмотров: 470;
